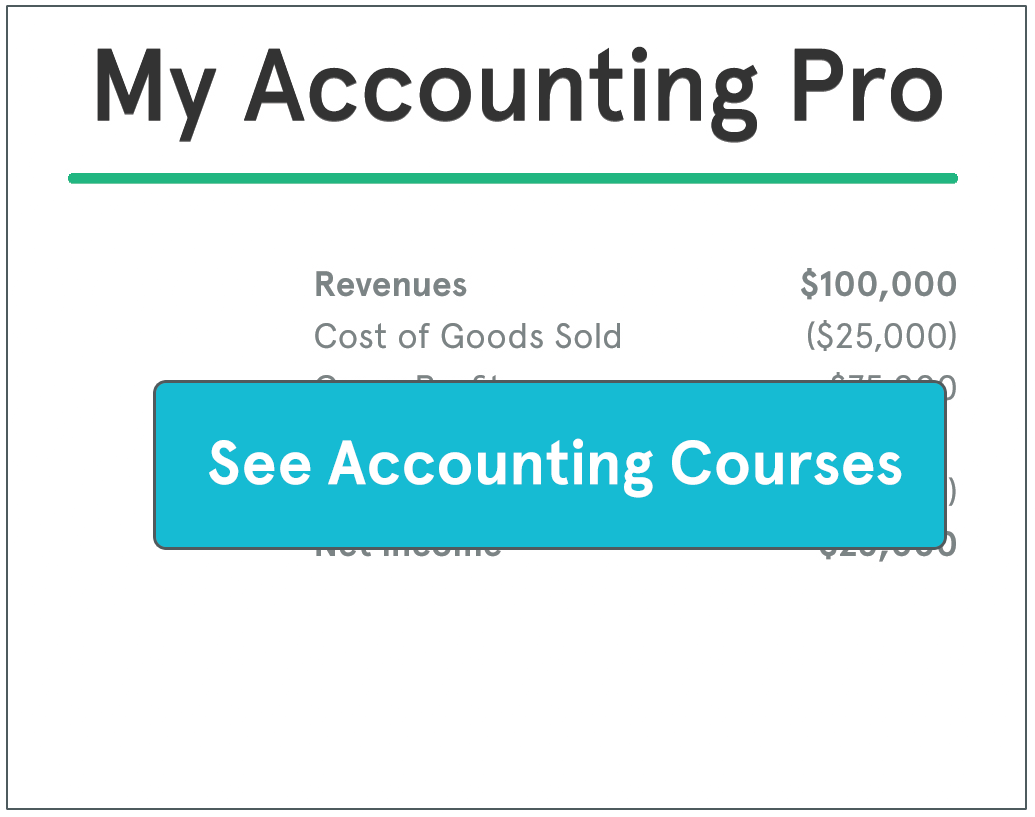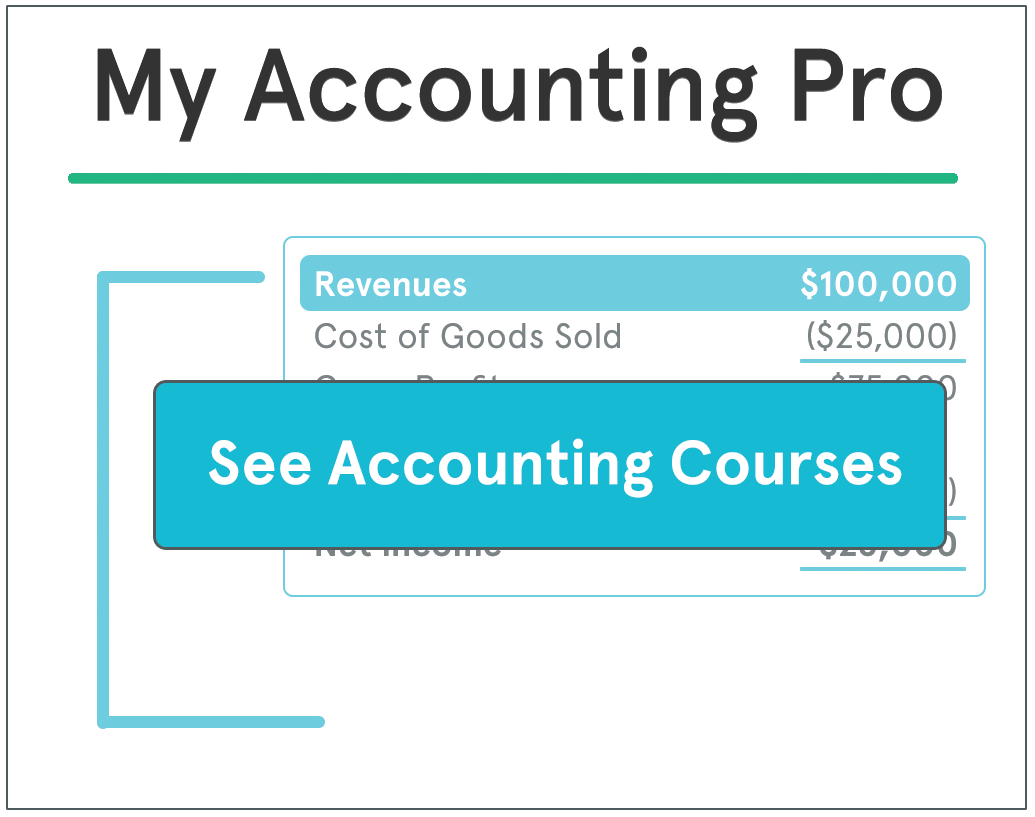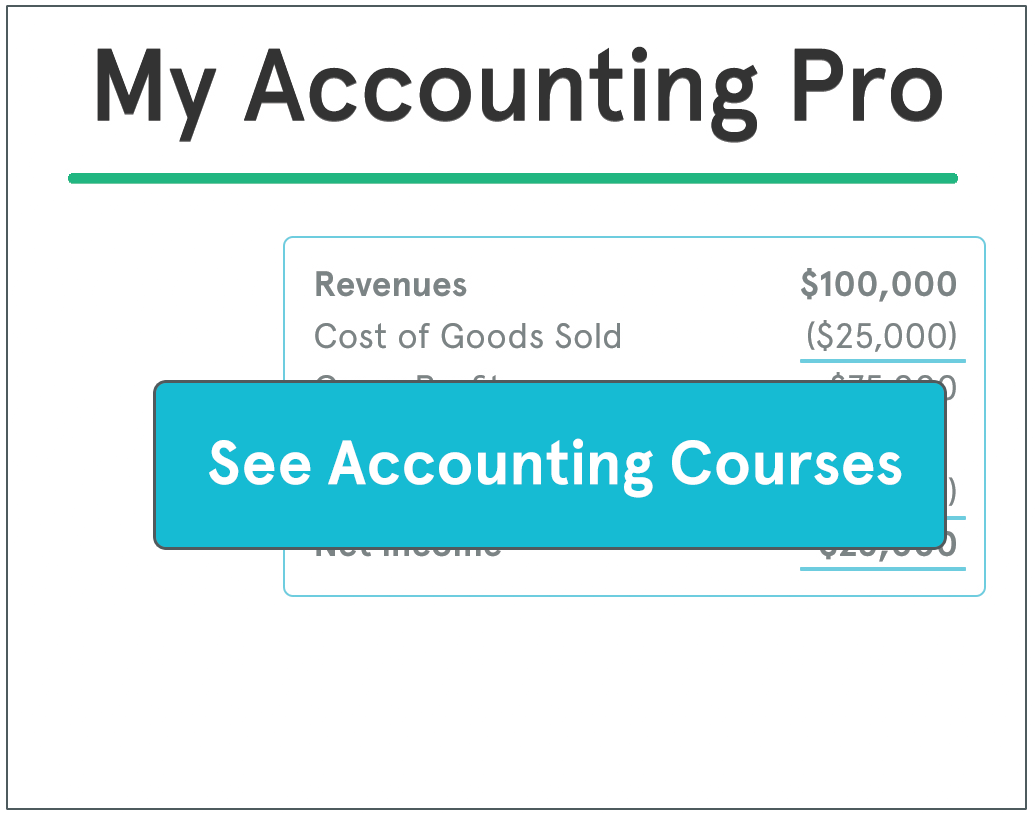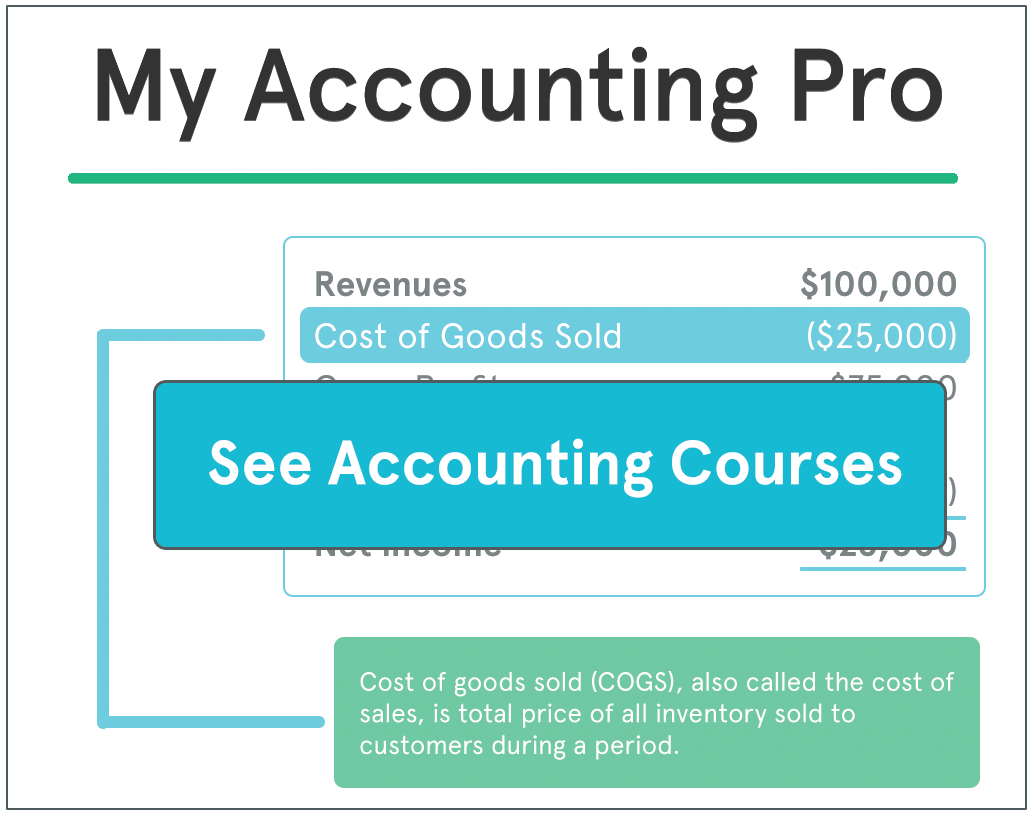
The Annual Percentage Rate (APR) is a calculation that estimates the percentage paid by a borrower or by an investment after any fees and additionally expenses involved are considered while the Average Percentage Yield (APY) is a calculation that incorporates the effect of compounding to estimate the cost of borrowing a loan or the return produced by a certain investment.
What is the Average Percentage Rate (APR)?
Contents [show]
The Annual Percentage Rate (APR) is a calculation that incorporates the impact of additional fees and transaction costs to the baseline interest rate, also known as Nominal Interest Rate, in order to give the borrower a better estimate of the actual cost of the debt.
Lenders in the United States are required by law to disclose the APR along with the Nominal Interest Rate offered for a particular loan, credit card or line of credit.
What is the Average Percentage Yield (APY)?
The Annual Percentage Yield (APY) is a calculation that is mostly employed to estimate the actual return produced by an investment considering the effect of compounding interest.
Nevertheless, it can also be used to understand the cost of borrowing if the borrower doesn’t intend to pay for the balance of the loan before it matures.
Key Takeaways
Definition and Calculation: APR (Annual Percentage Rate) represents the yearly interest rate charged for borrowing or earned through an investment without accounting for compound interest, while APY (Annual Percentage Yield) takes into account the effect of compounding interest over the year, showing the real rate of return or cost.
Impact of Compounding: APY provides a more accurate reflection of the actual earning potential or cost of a financial product due to its inclusion of compounding interest, whereas APR may underrepresent the total cost or return because it excludes this factor.
Usage in Financial Products: APR is commonly used to express the cost of loans and credit cards, offering a baseline rate comparison, while APY is often applied to savings accounts, CDs, and other investment products, highlighting the total interest earned or paid over a year.
APY vs APR Formulas
The formula to calculate both the Annual Percentage Rate (APR) and the Annual Percentage Yield (APY) are:
APR = [[(F + I + T) / P] / n] * 100
And;
APY = [(1 + r)n – 1] / n
Where:
F: The total fees involved in the operation during its lifetime.
I: The total interest charged or earned during the loan or investment’s lifetime.
T: The total transaction costs associated to the operation during its lifetime.
P: The principal amount of the loan or the
n: The number of years or time periods associated to the loan or investment.
r: The nominal interest rate paid or earned.
The result in both cases is expressed as a percentage and they can be compared among each other to understand the cost of borrowing or the potential profitability of the investment from different perspectives.

Key Differences between APY and APR
The Annual Percentage Return (APR) provides a more realistic measure of the cost of borrowing a loan as it incorporates the effect of any fees, transaction costs, and other expenses charged by the lender into the mix. On the other hand, the APR can also help in standardizing the cost of borrowing to allow the borrower to compare among different loans to pick the cheapest one.
Compounding Interest
Nevertheless, the APR fails to incorporate the effect of compounding interest into the calculation. This means that interest rates can ultimately be higher than the APR if the borrower doesn’t pay for the interest charged after each time period and, instead, lets it accumulate over time. The actual interest charge will be higher as the principal will be increased by the unpaid interest.
From the perspective of an investment, the APR could provide a better estimation of the percentage earned on a fixed income investment such as a Certificate of Deposit (CD) or a bond. By using the APR, the investor can deduct any fees involved in the transaction, even though the effect of compounding will not be considered. This latter characteristic of the APR makes it a less frequently used metric to analyze investment operations.
On the other hand, the Annual Percentage Yield, provides a better estimation of the potential profitability of an investment by considering the effect of compounding. If an investor decides to invest in a Certificate of Deposit that compounds on a monthly basis and the CD offers a nominal interest rate of 12% per year, the ultimate interest rate produced by the end of the year will be higher due to the monthly compounding feature.
Usage
Furthermore, the APY could also be employed for loans, yet the formula leaves out the effect of any fees or transaction costs associated to the instrument. While it incorporates the effect of compounding, the fact that it leaves out these important costs makes it a less reliable metric to estimate the cost of borrowing.
In most cases, the APR and the APY by themselves fail to portrait the actual cost of borrowing or the actual return earned on an investment and, therefore, while they are very useful, they should be analyzed along with other similar metrics to get a broader picture.
APR and APY Examples
Matthew is currently looking to invest money in a business a friend proposed to him. He doesn’t have the cash to make the investment but he is confident he can secure a loan from his financial institution and, therefore, he wants to estimate the Annual Percentage Rate (APR) charged by his lender with the potential Annual Percentage Yield (APY) produced by the investment to see if it would be profitable to finance the venture with a loan.
The amount of the investment would be $100,000 and his lender is charging an annual nominal interest rate of 6.54%. He also has to pay a 3% flat fee to take out the loan and transaction costs add up to $560 for the lifetime of the loan. The credit term offered by the lender is 36 months (3 years).
With this information we can calculate the APR for this loan as follows:
APR = [[($3,000 + $10,402 + $550) / $100,000] / 3] * 100 = 4.65%
The investment proposed to Matthew consists of a $100,000 invested that will be paid a nominal interest rate of 7% per year, compounded monthly. In this case the APY for this operation would be:
APY = [(1 + (0.07/12))(3 * 12) – 1] / 3 = 7.76%
This means that Matthew will earn a net return of approximately 3.21% per year on this operation as the APY produced by the invest is higher than the APR that he would have to pay for the loan.
Bottom Line
Calculating the APR manually is useful for investors, especially due to the fact that many lenders have found legal ways to exclude certain fees from the official calculation of the APR. Therefore, in some cases, lenders could underestimate the advertised APR by relying on these loopholes.
Frequently Asked Questions
How does APR differ from APY in terms of interest calculation?
APR represents the annual rate charged for borrowing or earned by an investment without compounding, while APY includes the effects of compounding interest annually, providing a more comprehensive measure of the actual interest rate.
Why is APY higher than APR when comparing the same financial product?
APY accounts for the compounding of interest within a given year, which can increase the total amount of interest earned or paid, making APY higher than APR for the same product.
Can APR and APY affect the total cost of a loan differently?
Yes, the APR provides the base interest rate of a loan, but the APY can show a higher cost due to compounding, especially in products where interest compounds more frequently than annually.
When comparing savings accounts, why should I look at APY instead of APR?
APY gives a more accurate representation of what you will actually earn on your savings due to compounding interest, making it a better metric for comparison than APR, which does not account for this effect.